Difference between revisions of "Energy resolution (ATLAS)"
(Created page with "Category:ATLAS Category:Event reconstruction Category:Electron/photon reconstruction ''This article only describes the situation for electrons and photons and cou...") |
(No difference)
|
Revision as of 15:28, 4 February 2020
This article only describes the situation for electrons and photons and could be expanded.
For electrons and photons in ATLAS, the relative energy resolution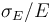 
of the electromagnetic calorimeter can be parametrised as
where the sampling-term coefficient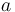 
, the noise-term coefficient 
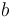and the constant-term 
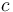are pseudorapidity-dependent parameters. The sampling term contributes mostly at low and intermediate energy and depends on calorimeter shower and sampling fluctuations. The noise term is dominated by the pileup noise in the standard LHC data taking (i.e. at an average number of interactions per bunch crossing of the order of a few dozen). At higher energies, the relative energy resolution tends asymptotically to the constant term, 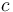, which in ATLAS has a design value of 0.7%. For electrons and converted photons, interactions with the material in front of the calorimeter also contribute to the 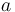and 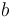terms.
A relative uncertainty of 10% on the sampling coefficient 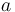is assumed based on test-beam data. The constant term 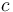is extracted from 
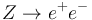measurements. In standard LHC data taking conditions, the energy resolution at low energy is dominated by the noise coefficient 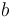and measuring the sampling coefficient 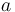to reduce its uncertainty is difficult.
